Believe it or, but there are people out there who haven't been following this whole government debt debate from the very beginning. (That is, whether locally financed debt can ever really be a burden to future generations since we effectively owe it to ourselves. ) After a recent link from Daniel Kuehn, I finally decided to bite the bullet and have a quick look over some posts. I may be covering very stale ground here, but please bear with me while I wade in at this very late stage.
Right, so following from Daniel's links, I've clicked through to Bob Murphy's blog. Bob is shameless about his addiction to this debt issue and apparently has infinity + 1 posts on it. I've read these two. (And didn't even make it to the comments!)
Bob neatly summarizes his (and Nick Rowe's) position via this nifty table, which shows an OLG endowment economy with 100 apples produced in each period and a 100 percent interest rate:
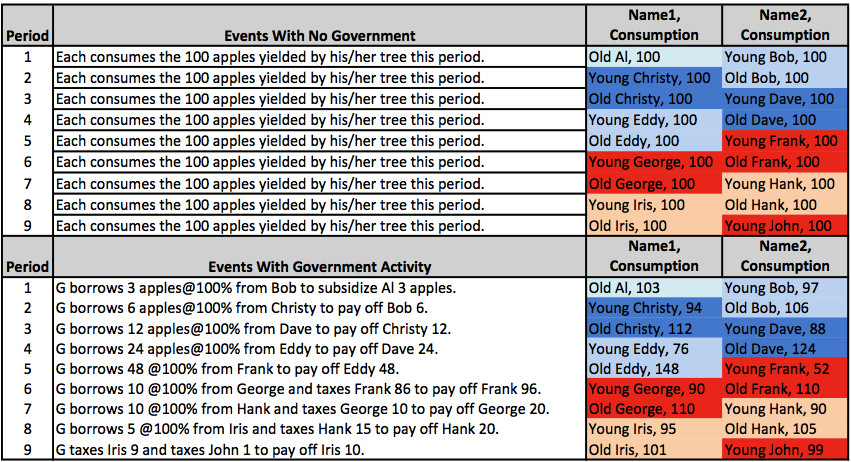
Using the above, Bob claims victory over people like Dean Baker and Paul Krugman, who have ostensibly been arguing that public debt cannot make an economy poorer if it is owed to its citizens. (We can see that all the people in red colouring from period 6 onwards are worse off.)
Nonetheless, I immediately have several questions upon seeing this table, including the obvious comment that you will always run into long-run problems if the interest rate exceeds GDP growth. Nonetheless, I'll stick to my most structural observation:
All the action effectively happens in period 6 when the government switches financing schemes. (In addition to young people financing old people, those same old people are now also being used to "pay back" their younger selves.)
However, it seems to me that Bob's model is missing the fundamental part of Baker et al.'s argument. Clearly, "old" Frank is not being compensated for the money that he is being taxed to pay back to his "younger self". In other words, it is the switching of financing schemes that matters. As I understand the Baker et al. argument, Frank should have 86 apples' worth of bonds in period 6 that must be left to someone when he dies. No-one inherits "Old" Franks' bonds and, consequently, they can never be redeemed.
Here is Baker making this exact point:
All the action effectively happens in period 6 when the government switches financing schemes. (In addition to young people financing old people, those same old people are now also being used to "pay back" their younger selves.)
However, it seems to me that Bob's model is missing the fundamental part of Baker et al.'s argument. Clearly, "old" Frank is not being compensated for the money that he is being taxed to pay back to his "younger self". In other words, it is the switching of financing schemes that matters. As I understand the Baker et al. argument, Frank should have 86 apples' worth of bonds in period 6 that must be left to someone when he dies. No-one inherits "Old" Franks' bonds and, consequently, they can never be redeemed.
Here is Baker making this exact point:
As a country we cannot impose huge debt burdens on our children. It is impossible, at least if we are referring to government debt. The reason is simple: at one point we will all be dead. That means that the ownership of our debt will be passed on to our children.
I think that a faithful representation of the Baker and Krugman argument would include the inheritance of bonds. In this case, from Old Frank to (say) Young Hank and so on. Of course, doing so here would mean that you run into a big problem because debt now exceeds total repayment ability. (i.e. 86 rolled over to period 7 at 100% interest is 174, which is obviously more than the 100 income available in any one period.) However, this is an entirely separate issue and again stems from the fact that this particular model assumes an interest rate of 100%, while GDP growth is zero.
As it stands, and with apologies Inigo Montoya, I'm not sure that this table means what people think it means.

Two quick things:
ReplyDelete==> There are 200 apples produced each period, not 100.
==> You're right, if people in the future are literally bequeathed the bonds from the previous generation, then they are OK (holding all other bequests constant). But what if the previous generation *sells* the bonds to them? Then they're screwed.
Nick Rowe has summarized this by saying that if you believe in Ricardian Equivalence, the old folks take steps to offset the burden that will fall on the next generations. But, Krugman has ridiculed Ricardian Equivalence on his blog. That's not the argument he was making, though you're right, he implicitly was assuming the bonds just get handed over to the next generation, without realizing they could be sold to them.
Hi stickman, you don't know me, but I am going to post here anyway, because this debate is very important to me.
ReplyDeleteThe "selling" versus "bequeathing" distinction is key. If there is any selling of bonds from one party to the next, which is virtually guaranteed in the real world, then there can be a net loss to everyone in the future.
My own solution to this great debate focuses on property titles, and tracks ALL property titles over time. Using this method,
we can identify losses with no corresponding gains.
DeleteBob and MF,
ReplyDeleteThanks for your comments and apologies for the delayed response. I don't want to come off like I'm a hotshot or anything[*], but I was up late last night drinking with a big name Chicago economist. I even made a joke about our hangovers in the morning. He laughed. We both did. It was awesome. (You should have been there.)
1) On the 100 vs 200 GDP, of course I agree. I was focusing on the production/repayment ability of any one individual, but should perhaps have been more precise with my terminology.
2) On bequests versus sale of debt, I think it better to write my response in a separate post. The summary version is that selling the bonds isn't what "screws" the future generations... Rather, it is (again) a simple artefact of the interest rate exceeding GDP growth. See here.
I have a couple posts on this that might interest you
ReplyDeleteFirst the the general argument about what Bob did wrong http://kenblogic.blogspot.com/2012/11/a-war-on-public-debt-and-paul-krugman.html
and then a reductio of Bob's criteria http://kenblogic.blogspot.com/2012/11/my-latest-salvo-in-ongoing-war-at-free.html
Thanks Ken.
DeleteYes, I agree that there is an argument to made about what Krugman actually meant by "worse off" (i.e. contemporaneous wealth of the economy versus what you have called the 'diagonal sections' across time periods). Again, I see this as largely being a function of differences between the interest rate (r) and economic growth (g)... Your point on the Sanatorum tax reinforces this view, since it is about how we can collapse a multi-period problem into a single-period problem.